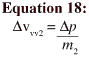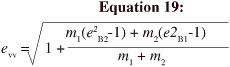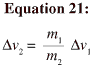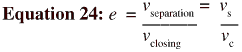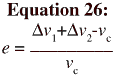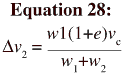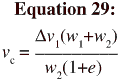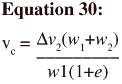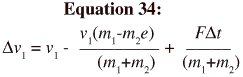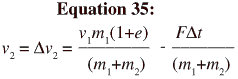Practical Reconstructions of LOSRIC
Now that we've discussed factors such as coefficient of restitution and conservation of momentum, as well as some practical ways of estimating speed changes in LOSRIC based on data obtained by numerous crash tests, we can start to put these discussions together in the form of a practical solution to the burning questions relating to injury causation and risk. The following is based largely on the work of Siegmund et al.3,5,12 and Szabo et al.13 Szabo and his colleagues have nicely codified much of this material.14
Siegmund et al.3 evaluated four methods for determining impact severity in low speed crashes: 1) the quasi-static isolator force method: using the force needed to begin isolator compression and, from this data, calculating acceleration and speed change; 2) the direct barrier method: here, the authors used data (isolator travel) from direct barrier tests to estimate vehicle speed change and, using the conservation of linear momentum, the other vehicle's speed change; 3) the MER method: determining the speed change based on barrier data in combination with momentum, energy and restitution; and 4) the staged collision, using actual or exemplar vehicles.
Clearly, number four is the most definitive method, but it is generally impractical due to the cost. They reported that method1 was the least accurate, while method3 predicted the actual speed changes of the vehicle-to-vehicle crash tests better than method2. However, a modified method2 most accurately predicted the actual speed changes.
Based on input of data and statistical analysis, higher order polynomials (in some cases, fourth degree polynomials) were developed for a best fit of the data. It was these equations which were used for method2. Again, they will not be valid when used for data outside the authors': specifically, the crash must not have been so severe as to bottom out the isolators.
They found that when both vehicles had isolators, direct use of sped change vs. isolator compression graphs for both vehicles could give two different speed changes for both vehicles. To solve the problem, they simply averaged the barrier-derived speed changes and then recalculated for each vehicle (recall that p=mv, so v=p/m):
where m1 and m2 are the masses of the vehicles, delta(vB1) and delta(vB2) are the barrier-derived speed changes for each isolator compression, delta(p) is the change in momentum, and delta(vvv1) and delta(vvv2) are the speed changes of the two vehicles in the vehicle-to-vehicle collision. This is the step added in the modified method 2.
In method 3, a crash restitution was calculated based on the following equation:
where evv is the collision restitution; m1 and m2 are the vehicle masses; and eB1 and eB2 are the barrier restitutions
The speed change of each vehicle can then be calculated as follows:
where:
delta(v1) and delta(v2) are the speed changes of the vehicles (m/s)
evv is the collision restitution
m1 and m2 are the vehicle masses (kg)
EB1 and EB2 are the energies absorbed by the barrier (J)
These barrier energies in low speed crashes are often equal to the isolator compression. One of the shortcomings of this method is that the derivation of evv assumes that the durations for barrier tests and vehicle-to-vehicle crashes are the same. However, experimental data fail to support this assumption.
Conservation of Momentum and Coefficient of Restitution Method
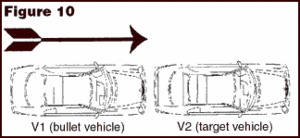
In LOSRIC, conservation of momentum equations can be used effectively, but it becomes important to consider the coefficient of restitution now because the collisions are rather elastic. Consider a typical aligned rear impact crash at low speed (see Figure 10). Recall that closing velocity (vc) is the equivalent of impact velocity in the case that the struck vehicle is stationary, or vc = v1-v2. Here, velocity is expressed in fps. The separation speed (vs) is the speed at which two vehicles separate, or vs = v2'-v1' (the prime symbol indicating velocities after the collision). From this, we can define the respective velocity changes as:
Figure 10: The bullet vehicle (V1) strikes the stopped vehicle target vehicle (V2). By tradition, the variables in the equations in this series of articles will refer to these vehicles by placing their respective numbers in subscript: the changes of velocity of V1 is written as v1, and the mass of V2 is written m2, for example. Reprinted with permission from Whiplash: The Masters' Certification Program, Module 2.
Recalling equation 12, and considering that in the case of momentum equations, we can substitute weight for mass, we get:
Recall the coefficient of restitution equation15. Szabo et al.14 have rearranged this in simpler English as:
To solve for e in terms of closing speed and changes in velocity, this can be rewritten as:
When I discussed the use of conservation of momentum equations in solving ACR problems earlier, recall that e was not considered. That is because when crashes result in crush damage, e is closer to zero and can be neglected. However, in LOSRIC - particularly in cases where little or no damage occurs - e must be considered, because it can be as high as 0.7 at speeds of 2.5 mph ad drops only to about 0.4 or so at speeds of 9 mph.5 In order to account for e, we can modify the conservation of momentum equations as follows (note: many schools of ACR do not teach this method, including - at least when I was there - Northwestern University's Traffic Institute):
You can see from these equations how a high value of e will nearly double the numerator. Now, if we happened to know the weights of the vehicles (easily obtained from published sources; see www.neptuneeng.comfor vehicle specifications), the velocity change of one vehicle, and the coefficient of restitution (which can also be crudely estimated from the observation of various crash tests - see references 3, 4 and 5 for more information), we could solve algebraically for the closing speed. For example, having estimated V1's speed change and e, the closing speed is:
Or, if it was V2's speed change that was known:
Once the closing speed is calculated, we could again algebraically solve for the other car's velocity change. However, in the majority of cases, we must use other methods because it is precisely the velocity change that we are actually trying to deduce.
Before proceeding to more practical methods, let's consider one more theoretical consideration rather unique to the reconstruction of low speed crashes: externally applied forces. Although this also includes second collisions, we're especially concerned with the effects of braking and the tires' rolling resistance. Both of these factors can have the effect of making the car virtually more massive and, since our calculations have considered mass as a variable, this can be important. Siegmund et al.5 found that braking in the target vehicle in LOSRIC effectively increased e from a value of about 0.6 to about 0.8. It also reduced the observed ranges of duration of the collision. The net effect was that the target vehicle's velocity change would drop, and the bullet's velocity change would increase by the same amount. It's been shown5 that even in low speed crashes where the target vehicle does not move, there is some velocity change in the car's body due to suspension compliance in pitch and surge directions.
Siegmund et al.5 accounted for the effect of braking by modifying the conservation of momentum equation in the following way:
where F1 and F2 are the braking forces, and delta(t) is the duration of the collision. The term Fdelta(t) represents an impulse, which is dependent on both the amount of force and its duration. In cases where a sloping surface is involved, the impulse terms can be rewritten, for example, as:
where O is the overall lockup factor, µ is the coefficient of friction, and f is the slope (+ for uphill). Combined, the form becomes:
Then, combining the external force-modified conservation of energy equation with the coefficient of restitution equation, and in the case of the target vehicle (V2) at rest, we have:
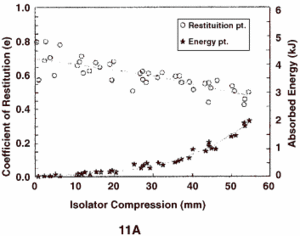
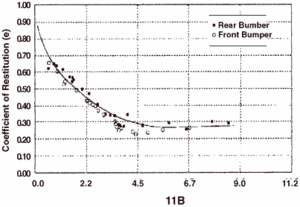
Figure 11 (11A and 11B) can serve as a practical gauge of the restitution behavior in LOSRIC.
Figures 11A and 11B. In A, the restitution behavior of a Volkswagen Rabbit is represented as a function of isolator compression. Adapted from Siegmund et al.3 In B, the restitution behavior of the bumpers of a Chevrolet Citation is represented as a function of velocity change and energy. Both were obtained from barrier tests and, of course, neither is necessarily representative of all other vehicles. Adapted from Siegmund et al.5 Reprinted with permission from Whiplash: The Masters' Certification Program, Module 2.
Having now covered this theoretical treatment of unbalanced external forces, let me point out that it is unlikely that braking will have a significant effect as an unbalanced external force because the pedal pressure will rarely be maintained after impact (see reference 4, p. 42 for a more in-depth discussion). It should be noted that Siegmund et al.5 used the parking brake and in some cases mechanically locked the brakes in order to simulate the effect of braking. This is likely to grossly overstate the effect of braking in real-world crashes.
Anderson et al.15 have also recently reported that braking had a negligible effect in low speed crashes in which the occupant was unaware of the impending crash. For that reason, I suggest that unless the driver attests firmly to a stout and uninterrupted application of the brakes, any calculations should omit the impulse concerning these forces. Moreover, in all but very low speed crashes - which would not likely result in injury in most cases - the rolling resistance of tires can be disregarded as negligible.
As noted earlier, Siegmund et al.3 have also discussed the method of combining conservation of momentum and conservation of energy (eq. 20 and 21), but the energy values must be derived from actual crash test data. Refer again to Figure 11.
In the final part of this series, Part V, we'll look at the application of techniques used in LOSRIC.
Click here for previous articles by Arthur Croft, DC, MS, MPH, FACO.