In this series of four articles, I will focus on the methodologies presently in use in ACT of LOSRICs, beginning with the basics and some necessary definitions and ending with the best methods in the final denouement. I might add that these methods are not taught in most schools of ACR. It would probably be a good idea to save these and re-read them all together when you've collected them.
Vehicle Dynamics
At the heart of ACR calculations lies the science of vehicle dynamics. The quantities used in vehicle dynamics include distance, time, velocity, acceleration, friction, drag and mass. We can calculate unknowns from these factors by knowing the values of other variables, but let's begin this section with some definitions. The reader will encounter a wide variety of equations, here and elsewhere, which require at some times metric (SI) units (kilograms, meters, etc.), and at other times Imperial units (pounds, feet, etc.). The science of physics is often shamefully exploited by we ACRs who knowingly but necessarily make frequent use of the educated guess in dealing with the problems of missing data. This is known as SWAG to many of you. Thus, I often think that we should have yet another set of units represented by symbols like a chicken wishbone, a light bulb, dice or a tiddlywink. Remember, the real units (SI and Imperial) are not interchangeable and care must be taken to use the correct units. Some equations call for SI units; others, Imperial units.
Distance. Very simply, distance refers to a body's change of position with respect to some fixed point. It is generally calculated from a vehicle's center of mass and is expressed in feet. Although a vehicle's center of mass can be calculated, I have omitted this step for the purposes of brevity. The symbol for distance is d (or, in some equations, s).
Time. Time really requires no special definition for our purposes and is expressed with the symbol t. Units of time are given in seconds, s or sec.
Velocity. Velocity (v) describes a body's rate of change of position with respect to time and is usually expressed as feet per second (fps or ft/sec). Technically, velocity is a vector quantity because it has both magnitude and direction. By convention, vector quantities are written in bold type face. Although we use these terms loosely and interchangeably, speed, in contrast to velocity, is a scalar quantity -- it expresses only magnitude (i.e., rate of travel). These values in fps can later be converted to the more familiar expression of mph by dividing by 1.47. The symbols used in vehicle dynamics equations are Vi for initial velocity and Ve for ending velocity. I will use the terms interchangeably here because in the concept of inline rear impact crashes, we assume the vector to be roughly the same in both vehicles.
Acceleration. Acceleration is also a vector. It describes a body's rate of change of velocity with respect to time and is generally expressed as feet per second squared or fps2. Acceleration always results from externally applied forces. The symbol used to represent acceleration in vehicle dynamics is a. More commonly, acceleration may be symbolized by g, which is equivalent to 32.2 fps2.
When thinking about acceleration, it's important to consider the relationship between change in velocity (deltaV) and acceleration. Neither by themselves are sufficient as gauges of injury potential. Astronauts, for example, may undergo deltaVs of 18,500 mph but do so over a long period. If we use the relationship (a)=deltaV/deltat, we can calculate that an astronaut undergoing a uniform deltaV of 18,500 mph (27,195 fps) over a span of one hour would be subjected to an average of 7.6 fps2 or 0.2g. A stationary vehicle struck from the rear and accelerated to 5 mph (7.4 fps) in a span of 0.12 seconds is exposed to an average acceleration of 1.9g. However, this figure represents the average acceleration of the vehicle as indicated by the angle brackets. The peak acceleration, clearly a more important factor as it relates to injury potential, is usually much higher. And we know from the many human volunteer crash tests that have been reported, as well as from our own crash tests, that the peak occupant head and neck accelerations can be multiples of those of the vehicle. In such a collision, measured at the head, acceleration can be in excess of 12g.
Coefficient of friction. The coefficient of friction is a ratio of the horizontal force required to move an object across a surface and the vertical force (or weight) of the object. Since it is expressed as a fraction, there are no units. The symbol used to represent the coefficient of friction is u.
Drag factor. Drag factor (f) describes the acceleration of a body (which can have either a positive or negative value) as a decimal fraction of the acceleration due to Earth's gravity (g). One g, the acceleration from gravity on Earth, is 32.2 fps2.
Mass. Mass is a measure of the inertia of the body. The more mass a body has, the harder it is to change its state of motion. Mass is expressed in kg or lb, and the symbol we will use for it is m. However, I should pre-empt a spate of e-mail from you physics majors and former engineers by acknowledging that mass is not the same as weight. This value (m) can sometimes be confusing. Recall that Newton's second law of motion states that force, F, is equal to the product of mass, m, and acceleration, a:
Equation 1: F = ma
The force of an object in free fall is its weight, w. The acceleration, a, is equal to the acceleration of gravity, g. By substituting the expression F=w and a=g, we arrive at a new equation:
Equation 2: w = mg
By solving algebraically for m, we arrive at:
Equation 3: m = w/g
Therefore, since the acceleration of Earth's gravity is one g, for practical purposes, we can consider m and w equivalent. (This is allowable in pure momentum equations, but we can't get away with it when considering externally applied forces, as we'll see later.) Looking at things more precisely -- which we don't do often enough -- a 4,000-pound car has a mass of 125 lb/fps2 (4,000 lb/32 fps2).
Moment of force. The moment of force, M, is a measurement of the extent that the force, F, tends to cause a body to rotate around an axis. Moment of force is defined as the product of the force and the length of the moment arm, d (distance). The perpendicular distance from the pivot point to the line of action of the force describes the moment arm. This is expressed by the equation:
Equation 4: M = Fd
Center of mass and center of gravity. For rigid bodies, these are equivalent and, for the purposes of ACR only, we will allow them to be used synonymously. If you balance a baseball bat (for which the center of mass might not be easily guessed) so that it lies horizontally balanced across your finger, you've found its center of mass. In ACR, we generally consider a car's center of mass to be an imaginary point at which lines drawn from corner to corner cross (from a top view).
Newton's Laws of Motion
The physics of ACR is really quite simple and requires no more than an understanding of Newton's three fundamental laws of motion.
Newton's First Law of Motion. Newton's first law of motion states that a body will remain at rest or, if in uniform motion (constant velocity in a straight line), will remain in motion unless it is acted upon by an outside force. Simply put, force is an action that changes the motion of a body.
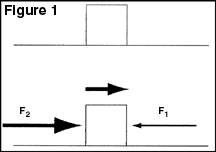
[Figure 1. Newton's first law of motion. If force2 > force1 (unbalanced force), net motion occurs. Reprinted with permission from Whiplash: The Masters' Certification Program, Module 2.]
Newton's Second Law of Motion. In his second law, Newton defined what the change in motion predicted from his first law would be. The law states that if the net force acting on a body is not zero, the body will be accelerated in the direction of the net force and that the magnitude of that acceleration will be directly proportional to the net force and inversely proportional to the mass of the body. This law is frequently expressed by equation 1, F = ma; force is equal to mass times acceleration. It can be rewritten as:
Equation 5: a = F/m
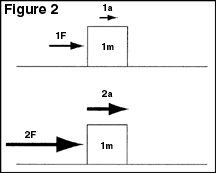
[Figure 2. Newton's second law of motion. Acceleration is directly proportional to the net force (a = F/m) and inversely proportional to the mass. F = force; a = acceleration; m = mass. Reprinted with permission from Whiplash: The Masters' Certification Program, Module 2.]
Newton's Third Law of Motion. Newton's third law of motion states that whenever a body exerts a force upon another body, that other body exerts a force equal in magnitude, but opposite in direction, upon the first body. This third law is conceptually the most difficult for most people to understand. Restated in familiar terms, "For every action, there is an equal and opposite reaction." It tells us that when two vehicles collide, each will exert and equal but opposite force upon the other.
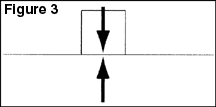
[Figure 3. Newton's third law of motion. Reprinted with permission from Whiplash: The Masters' Certification Program, Module 2.]
Relationships between Quantities
Coefficient of Friction and Drag Factor
When tires skid on the roadway, we must consider the coefficient of friction (u) and the drag factor (f). When all four tires are skidding on level pavement, the coefficient of friction and the drag factor are equal. In that case, f = u. However, many times the skidding occurs on a grade. In that case, we must consider either an uphill grade or downhill grade, in which case the equation can be rewritten respectively either as:
Equation 6: f = u + G
or
Equation 7: f = u - G
When all tires are not skidding -- a common situation -- we must calculate what is known as the resultant drag factor. This can be expressed as the equation:
Equation 8: fR = [ff - xf (ff-fr)]/[1 - z (ff-fr)]where:
• fR is the resultant drag factor
• ff is the drag factor on the front axle
• fr is the drag factor on the rear axle
• xf is the longitudinal location of the center of mass from the front axle as a decimal fraction of the wheel base • z is the height of the center of mass as a decimal fraction of the wheel base
The derivations of xf and z are omitted here for the sake of brevity. As we'll see later, in most cases of LOSRIC, the effects of braking are negligible. However, when skid marks are available for measurement, these formulas can be important in developing ranges of speed.
With a higher center of mass, the weight of a vehicle will shift forward during heavy braking (negative a) and place more weight on the front tires and less on the rear. The resultant drag factor can be used to calculate the amount of weight shifting that occurs during heavy braking. However, in our application of LOSRIC, we will not be concerned with these equations.
Drag Factor and Acceleration
In our definition of the coefficient of friction, u=F/w, if we assume that f=F/w or F=fw, and recall that Newton's second law of motion gave us F=ma and m=w/g, we can derive the equation F=(a)w/g and then simplify this equation to f=a/g or:
Equation 9: a = fg
These relationships will be important later in our calculations of velocity.
Other Equations
The motions and actions of vehicles can be quite complex and present special problems for the ACR. For example, a car may skid for some distance on the roadway.
Then, leaving the roadway, it may skid for some additional distance in loose gravel on a downgrade and then fall several feet as it crosses a culvert and strikes the upgrade of the culvert and stops. There are special equations to account for flips, vaults, falls and sideslip critical velocity, but again, we will not be concerned with those special situations with LOSRIC. The reader is referred to reference 1 for information about these specific situations.
Vehicle Dynamics Example
Now lets's take a practical example of how a calculated resultant drag factor for a particular roadway can be useful in determining a vehicle's initial velocity when a measured set of skid marks are present. Suppose that we had calculated a drag factor of 0.56 using equation 8 above, and that 36 feet of skid marks were measured. To calculate the vehicle's initial speed, the equation we would use would be:
Equation 10: Vi = (square root) Ve2 - 2ad
where Vi is initial velocity and Ve is ending velocity. Substituting, we would have:
Equation 10a: Vi = (square root) (0)2 - 2(0.56)(-32.2)(36)
or 36 feet per second (fps) or 24.5 mph. Notice that the acceleration gets a negative sign since it is, essentially, deceleration. This solves the problem posed by attempting to take the square root of a negative value. Factors that limit the accuracy of this figure include the possibility that some pre-skid braking occurred, in which case the initial speed would have been higher. Thus, the figure calculated will be conservative. Note that you can also reverse Ve and Vi. I recently had a case in which I was called upon to write a rebuttal to an engineer's ACR report. The case involved a van which had backed up into a car. To estimate the closing velocity (Ve), I used equation 10b:
Equation 10b: Ve = (square root) (0)2 - 2(-5)(5) = 7.1
In this case, I am allowing for an approximate acceleration from a dead stop of 5 fps2. In a forward motion, this will generally be more in the range of 10 fps2. The distance between the two vehicles was reported to be 5 feet.
Thus, the approximate closing velocity (vc) was 7.1 fps. Using the new symbology I suggested earlier, we could say it is OK to call this value 7.1 fps or vc (hand) = 7.1 fps!
That's all for now. The best is yet to come. In Part II, we'll discuss conservation of momentum and the coefficient of restitution.
References
1. Fricke LB. Traffic accident reconstruction. Volume 2 of the Traffic Accident Investigation Manual, Evanston, Northwestern University Traffic Institute, 1990, sec. 68, p. 27.
Click here for previous articles by Arthur Croft, DC, MS, MPH, FACO.