In Part I (see the May 31, 1999 issue of DC), we discussed a few definitions and looked at a couple of applications of vehicle dynamic equations useful in reconstructing low speed rear impact crashes (LOSRIC).
Conservation of Momentum
Newton expressed the quantity of motion (now called momentum, and expressed as p) as the product of mass and velocity. In fact, originally, his second law was expressed in terms of momentum rather than acceleration. The momentum is:
Equation 11.
p=mv (or p=wv)
The law of conservation of momentum states that in any group of objects that act upon each other, the total momentum before the action is equal to the total momentum after the action. Thus:
Equation 12.
m1v1+m2v2 = m1v1'+m2v2'
The prime sign (') indicates the after-accident condition. And remember that in accident reconstruction, we often consider weight (w) to be synonymous with mass (m) for practical purposes. However, we generally do not add the weight of the vehicle load -- such as passengers, luggage, etc. -- to that of the vehicle because they are not technically attached to the car and thus are not a part of the car's mass. For example, at impact, these objects will move freely within the car. Moreover, you'll find that the results of many of the vehicle dynamics equations are not changed markedly anyway by adding the weights of, say, two passengers. Arguably though, when passengers are well restrained in the car, their mass does contribute to the overall crash dynamics. Now let's take a look at how the vehicle's weight can affect the collision. Consider first a head-on collision between two cars (V1 and V2) which have different masses, different pre-impact velocities, and stay together after impact, where:
Given:
w1 = 5000 lb
w2 = 2500 lb
v1 = 20 fps
v2 = 40 fps
v1w1+v2w2 = v1'w1+v2'w2 or v1w1+v2w2 = v'(w1+w2), so 20(5000)+(40)(2500) = v'(5000+2500) v' = 0
This collision resulted in zero net momentum after-impact velocity. The higher speed of vehicle two (V2) was cancelled out by the greater weight of vehicle one (V1).
Now let's see how this equation can be applied to same-direction collisions. Suppose this time, a stationary vehicle (V2) is struck from the rear by another car of the same size (V1), and we know from vehicle dynamics calculations that the ending velocity of the two cars (i.e., after impact) was 45 fps (30.6 mph). Here is how we would calculate the impact velocity of V1:
Given:
w1 = 5000 lb
w2 = 5000 lb
v' = 45 fps
v2 = 0 fps
v1w1+v2w2 = v1'w1+v2'w2 or
v1w1+v2w2 = v'(w1+w2), so
(v1)(5000)+(0)(5000) = 45(5000+5000)
v1 = 90 fps (61.2 mph)
By now, it should be clear that to calculate beginning or ending velocities with any degree of accuracy, we must begin with the following necessary information:
- The approach path of each vehicle to impact. This can be determined from tire prints or skid marks, or an analysis of damage to the vehicles.
- The departure path of each vehicle. Tire prints, skid marks, gouges and grooves in the roadway, a liquid dribble path, or the final resting place of vehicles can provide this information.
- The weight and load of each vehicle.
- The post-impact velocity of each vehicle. This can be derived from vehicle dynamics equations (an example of which was provided earlier).
In real-world ACR scenarios involving LOSRIC, the ACR is frequently furnished with only photographs of vehicles that show little or no damage. Police scaled diagrams are usually not available, and pre- and post-crash motions and velocities can only be crudely and unreliably estimated from eyewitness accounts (which commonly conflict when there are more than one!), or the unquestionably biased accounts of the involved parties which, aside from the potential of bias, are also known to be unreliable. This is where the science of ACT begins to break down. While most ACRs are honest and will freely admit the limitations of their craft in such circumstances, there are some who can't resist the temptation to apply liberal amounts of "fudge factor," facing their clients, and opposing attorneys, with pretensions of scientific exactitude. Theirs is a "science" machine operating at the hairy edge of reality, fueled mostly by intuition and guesswork, and churning out copious amounts of hyperbole and prevarication. One of the first signs of this type of work will be the absence of calculations in the report, with the findings (deltaV, etc.) presented with single figures (as opposed to a range) with pretentious accuracy to the decimal place. Where a reasonable report would give the deltaV as "from 2-5 mph," these phonies will say, "The deltaV was 2.4 mph." This degree of accuracy is never possible.
Parallelogram or Vector Analysis Solution of Conservation of Momentum Problems
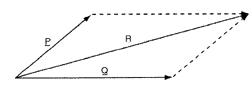
A way of solving momentum equations in which the cars do not behave and remain inline is to use a parallelogram or vector analysis. The parallelogram law states that two vectors may be replaced by a single vector called a resultant R, obtained by drawing the diagonal of the parallelogram which has sides equal to the given vectors (see Figure 4).
[Figure 4: Vector analysis method. P = the post-collision momentum for V1; Q = the post-collision momentum for V2; R = the resultant, or total post-collision momentum. With permission from Whiplash: The Masters' Certification Program, Module 2.]
To solve this type of problem, we first must have crated a scaled accident diagram and reconstructed the accident. Having determined the position of maximum engagement, we can then determine the first contact position. The development of such collision diagrams is well beyond the scope of this series of articles, but provides us with the basis of the angles in the givens in the examples below. The post-impact velocities (v') are derived from vehicle dynamics equations.
Given:
w1 = 3500 lb
w2 = 2500 lb
v1' = 30 fps
v2' = 30 fps
Theta1' = 40 °
Theta2' = 25°
Theta2 = 10°
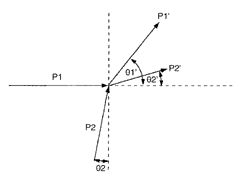
We now know the approach and departure angles for the two vehicles (V1 and V2). By convention, the left side of the x coordinate will represent the pre-impact momentum (P1) of V1 (see Figure 5).
[Figure 5: Pre- and post-impact (primed) momenta vectors for V1 and V2. See text. With permission from Whiplash: The Masters' Certification Program, Module 2.]
P2 will be the pre-impact momentum of V2. P1' and P2' are the momenta of the respective vehicles after the crash. From equation 11, we make the following calculations:
- P1' = w1v1'; P1' = 3500(30) = 105,000 lbs fps
- P2' = w2v2'; P2' = 2500(30) = 75,000 lbs fps
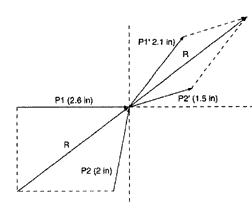
- Using an arbitrary scale of 50,000 lbs fps/1 inch, we calculate a line length of 2.1 inches (in) for P1' (P1' = 105,000/50,000 = 2.1 in), and 1.5 for P2' (P2' = 75,000/50,000 = 1.5 in).
- Using these lengths, we complete the parallelogram (see Figure 6), and draw the resultant R. We then move R back into the region of P1 and P2, which in this case are 2.6 in and 2.0 inches respectively.
- Now we can calculate the momenta of P1 and P2. P1 = 2.6(50,000) = 130,000 lb fps; P2 = 2.0(50,000) = 100,000 lb fps. This can be converted to velocity based on the relationship v = P/w: v1 = 130,000/3500 = 37.1 fps (25.2 mph), and v2 = 100,000/2500 = 40.0 fps (27.2 mph).
As you might guess, the inherent accuracy of this approach is dependent on the accuracy of the collision diagram, primarily, but also on the accuracy of the subsequent measurements. In practice, a room full of experienced ACRs will come up with a range of figures +/- a few mph at best. Of course, this usually has nothing much to do with LOSRIC, but before we leave the topic, there are still other ways to solve momentum problems.
[Figure 6: Vector solution to law of conservation of momentum problems. With permission from Whiplash: The Masters' Certification Program, Module 2.]
Mathematical Solution of Conservation of Momentum Problems
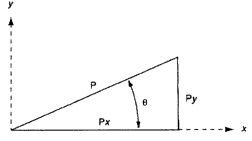
In addition to graphic solutions for momentum problems, we can use mathematical solutions. The same input data must be known in either case. Any vector quantity, such as force, velocity or momentum, can easily be resolved into rectangular components. In Figure 7 below, vector P has been resolved into x and y components. These would be as follows:
Px = P cos;
Py = P sin;
The vector P can be resolved into its x and y components as long as the angle 0 is known.
[Figure 7: The vector P can be resolved into x and y components, Px and Py, if the angle 0 is known. With permission from Whiplash: The Masters' Certification Program, Module 2.]
To solve the problem presented below mathematically, we resolve the pre- and post-collision momentum vectors into their x and y components. These components for both pre- and post-impact velocity are set to equal each other and the pre-collision velocities are then solved for. The equations then become:
Equation 13.
v1 = (v1'w1cos1'+v2'w2cos2) / (w1cos1)
Equation 14.
v2 = (v1'w1sin1'+v2'w2sin2') / (w2sin2)
We could then solve the type of problem shown in Figure 4 without having to draw parallelograms. However, once again the reader is cautioned that the output is only as good as the input, and the accuracy of the input is often questionable. In such instances, an ACR must be conservative and calculate a range of answers based on a range of possible input. As Lynn B. Fricke, the author of Traffic Accident Reconstruction, Volume 21 wisely cautioned: "You might start to believe your answers are more accurate than they actually are, forgetting that many of the inputs are only slightly better than guesses. Clearly, this is a time to exercise caution and understand the limitation of your analysis."
Conservation of Momentum "Difficulties"
Newton's laws of motion are precise. Looking at the conservation of momentum relationship in more familiar terms, if a billiard ball strikes a stationary ball, and the two balls then move off at different velocities, we could say that the combined momenta of the two balls after the impact is equal to the first ball's original momentum. In car crashes, this relationship is clouded by an apparent loss of momentum which results from energy lost; for example, into the ground when two cars collide or, at the submicroscopic level, with the breaking of bonds. Other forms of (probably negligible) energy loss include losses via heat transfer and sound. In cases where energy loss is significant, other methods of reconstruction must be relied upon. One of the reasons to include a restitution factor in reconstructions of low speed crashes is to account for apparent losses of momentum. For example, Szabo and Welcher2 reported the results of their crash testing of Ford Escorts. Careful examination of their data revealed an apparent loss of momentum of 15%, for which they provided no explanation or discussion.
Coefficient of Restitution
In low speed crashes, this coefficient of restitution e becomes important. The relationship is illustrated in the following equation:
Equation 15.
e = (VF'-VR') / (VF-VR)
This is essentially a ratio of the vehicles' (F=front; R=rear) post-impact velocities (primed values) and their pre-impact velocities: the rebound or restitutional speed divided by the deforming or impact speed. Thus, it's related to the kinetic energies involved in crashes. The value of e is always negative but, for shorthand purposes, we usually do not sign it that way. When the value of e is closer to zero, it is said to be a plastic type of collision, and when the value of e is closer to one, the collision is mostly elastic in nature. In truth, there are no perfectly elastic materials (there is always some energy of deformation that can't be returned). If there were, a perpetual motion machine would become possible.
Another way of thinking about this value is that it represents the springiness of a collision. A body is said to have elastic properties if the deformation caused by the induction of force is recovered completely after the force is removed, and the energy stored by the body during its deformed state is also recovered. When deformation and energy are not restored, the collision is plastic.
In reality, we generally consider these things by degree, since pure plastic and elastic responses don't occur. In the case of the two colliding billiard balls, the collision was quite elastic and e would be close to one. If, however, the two balls were made of soft wax, they would deform on impact in a plastic flow and e would be closer to zero. When the sheet metal and other deformable parts of the car are permanently bent, the collision is likewise more plastic in nature (although some of this deformation will be reversed after the crash due to the springiness of steel -- the difference between dynamic and static crush), and less of the impact energy is transferred to the occupants. Conversely, in an elastic crash, a large portion of the collision energy is transferred to the occupant, thereby increasing the potential for injury.
Complicating matters somewhat, the value of e varies over a range of crash velocities such that at very low velocities (e.g., 1-2 mph deltaV), e is very high (0.8 or so), and at higher velocities (e.g., 9 mph and above), it may be as low as 0.1 or less. Therefore, while it is reasonable to ignore restitution in the reconstruction of higher velocity crashes, it is not OK in LOSRIC.
Click here for previous articles by Arthur Croft, DC, MS, MPH, FACO.